comet P/2019 LD2 (ATLAS)

In this mpml message, Sam Deen pointed out that this asteroid in not a Jupyter Troian  but probably a 4-8 km size long period comet that in February 2017 achieved a perihelium low enough to start outgassing .

In this simulation, Tony Dunn confirmed that 2019 LD2 is not a Troian, Greek or Hilda but a comet that regulary makes very close passed to Jupiter and Saturn.

In fact, the object has now been given a cometary designation P/2019 LD2 (ATLAS)

For what's worth due to the uncertainty, I also tried a couple of day ago  to see when the comet entered the solar system assuming a conventional distance greater than 100 au .

I simulated 100 clones in the past 280K years (Mercury6 software by John E. Chambers - Bulirsch-Stoer integration, intial timestep 0.05 days).

	Clones			Target
	mean	sd		mean	sd
q	4.57802477541	1.671146e-05		4.57801992309	1.6782e-05
e	0.13528015709	9.88572e-06		0.13527811197	9.8665e-06
i	11.55192320685	1.746557e-05		11.55192194572	1.7512e-05
peri	123.44833869054	0.00209362353		123.44893096687	0.0021043
node	179.74537221523	0.00017704315		179.74536895343	0.00017777
tp	2458949.96748775	0.01979864266		2458949.97292011	0.01993

**In the meantime, the nominal parameters have been updated but I hope that this does not radically change the macroscopic behaviour.

The nominal comet entered the solar system about 32K years ago, while the most recent date for a clone to enter the solar system was about 4K years ago.

This is the overall time distribution (being a backward simulation, the clones are "ejected" from the point of view of the simulator...):

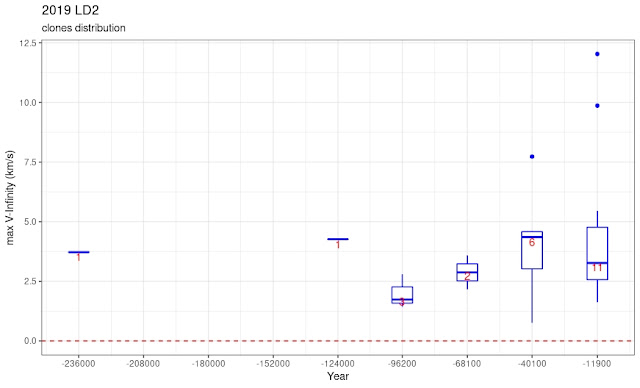
At first glance, it seemed to me that some clones entered the solar system on a hyperbolic trajectory (total energy > 0) but this is not the case.

This would be quite strange if real, many thanks to Marshall Eubanks for noting this aspect.

Looking better, we see situations like this, where you can see that the simulation does not end with the clone on a hyperbolic trajectory even if that orbit was apparently achieved in a given moment:

Time	a	e	i	peri	node	long
311100.5	11.10955	0.56037	12.7835	207.0631	179.7592	26.8223
311000.5	12.17985	0.599077	13.1885	206.6135	180.4985	27.112
310900.5	-6.12701	1.813375	2.8379	213.9255	160.4411	14.3666
310800.5	126.90366	0.960378	4.4906	347.4277	20.2166	7.6443
310700.5	58.4381	0.913926	4.4251	347.0157	20.7539	7.7696
310600.5	48.95964	0.897233	4.3941	346.7163	21.1435	7.8598

The reason why this can happen was explained by Jean Meeus:

"When one calculates the HELIOCENTRIC orbit near the time the object is close to Jupiter, one obtains a hyperbolic orbit, that however is meaningless because at that instant the object is attracted more by Jupiter than by the Sun."

So in the following plots, it is safe to ignore the brief moments where the orbit has apparently a total energy > 0 

This is the v_infinity distribution:   plot not meaninfgful (it just "captures" a brief close encounter moment):

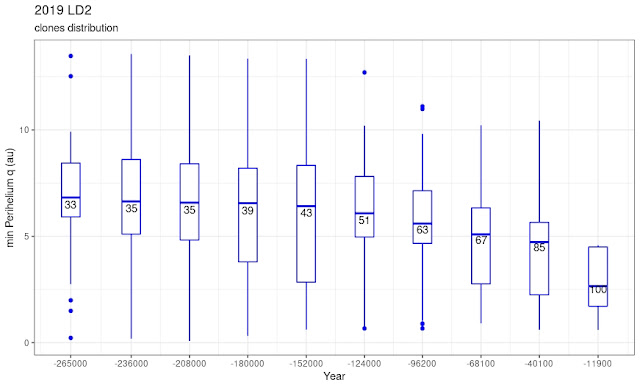
Mimimum perihelium distribution

Due to the wild uncertainty, the minimum perihelium could have been very low: in fact, about 10% of the clones could have had a close pass with Earth but not sooner than 4K years ago.

Max eccentricity distribution

(dis-regard the points with eccentricity > 1)

Kind Regards,

Alessandro Odasso